Minimal Convex Imaginary Cubes

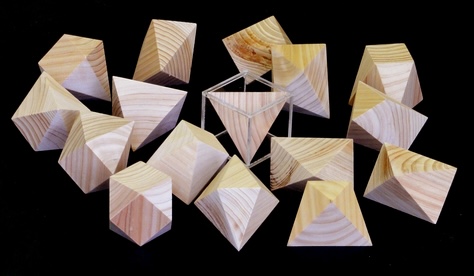
There are infinitely many convex polyhedra that qualify as Imaginary Cubes, such as the regular tetrahedron, H-shape, and T-shape. Among them, the most fundamental ones—called the minimal convex Imaginary Cubes—can be classified into 16 types. Specifically, if we require that the vertices lying on the edges of a cube come to the midpoints of those edges when placed inside a cube-shaped box, there are exactly 16 such polyhedra.

These 16 sets were crafted by Mr. Hiroshi Nakagawa, a master of wooden polyhedral craftsmanship.

When these polyhedra are presented on their own, it is not easy to realize that they are Imaginary Cubes. However, once placed inside a transparent acrylic cube and viewed from three orthogonal directions, their nature becomes instantly apparent.

The paper models of the 16 Imaginary Cubes shown below were first created by Kei Terayama, who was a student at the time.

• Created: January 2010 (Paper models: July 2007)
• Special Thanks: Hiroshi Nakagawa, Kei Terayama